Electric heating tubes are a common type of electric heating equipment widely used in various industrial and domestic environments. Accurately calculating the heat flux density (q value) on the surface of an electric heating tube is crucial because it directly affects the efficiency and safety of the tube. By analyzing the temperature map of a powered electric heating tube, one can effectively determine the corresponding q value. This article will detail this process, including the necessary theoretical foundations, data acquisition, and processing methods.

I. Theoretical Foundations
Calculating the heat flux density q requires an understanding of the basic principles of heat transfer. The heat transfer on the surface of an electric heating tube mainly involves conduction and convection. Conduction is the transfer of heat within and on the surface of the tube, while convection is the heat exchange between the tube surface and the surrounding air.
According to Fourier’s law, thermal conductivity can be expressed as:

In practical applications, the heat flux density q of an electric heating tube is usually solved by considering both conduction and convection heat transfer.
II. Acquisition of Temperature Maps
To determine the q value from the temperature map of an electric heating tube, precise temperature data must be obtained. Here are some common methods for acquiring temperature maps:
1. Thermal Imaging Technology
A thermal imaging camera is an effective tool that can obtain the temperature distribution on the surface of an electric heating tube in real time. The camera detects surface temperature through infrared radiation and generates temperature images.
2. Thermocouples
Thermocouples can accurately measure local temperatures on the surface of an electric heating tube. By placing multiple thermocouples on the tube surface, a detailed temperature distribution map can be created.
3. Thermistors
Thermistors change their resistance value with temperature changes. By measuring the resistance, the corresponding temperature can be calculated. Arranging multiple thermistors on the tube surface can also provide a detailed temperature distribution map.
III. Processing Temperature Data
After obtaining the temperature map, the data must be processed to calculate the heat flux density q.
1. Calculating the Temperature Gradient
For each temperature point, calculate the gradient of the surrounding temperatures. This can be achieved using numerical differentiation methods. For example, for a two-dimensional temperature distribution, the gradient can be calculated using the following formulas:

2. Determining Heat Transfer Coefficients
The thermal conductivity \( k \) and convective heat transfer coefficient \( h \) are characteristic parameters of the material and environment, which can be obtained through experiments or literature. With these parameters, Fourier’s law and Newton’s law of cooling can be applied to calculate the heat flux density q.
3. Comprehensive Calculation
Combine the temperature gradient and heat transfer coefficients and apply the aforementioned conduction and convective heat transfer formulas to calculate the heat flux density q at each temperature point. Then, by integrating these values, the average heat flux density of the entire surface can be obtained.

IV. Example Analysis
Assume we have obtained the temperature map of a powered electric heating tube using a thermal imaging camera, as shown in Figure 1. The temperature distribution is clearly visible. Given the thermal conductivity \( k \) of the tube material and the convective heat transfer coefficient \( h \) of the environment, we can follow the steps outlined above for the calculation.
First, use numerical differentiation methods to calculate the temperature gradient, then substitute these gradients into Fourier’s law and Newton’s law of cooling to obtain the heat flux density q at each temperature point. Finally, calculate the average heat flux density of the entire surface.
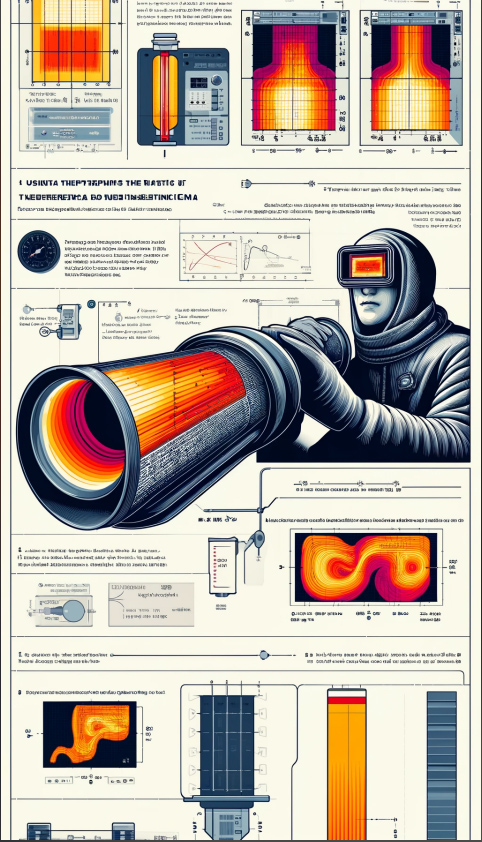
By detailed analysis and calculation of the temperature map of a powered electric heating tube, the heat flux density q can be accurately determined. This process requires a solid theoretical foundation in heat transfer, precise measurement techniques, and data processing methods. Accurate calculation of the q value of an electric heating tube is essential for optimizing its design and improving operational efficiency.